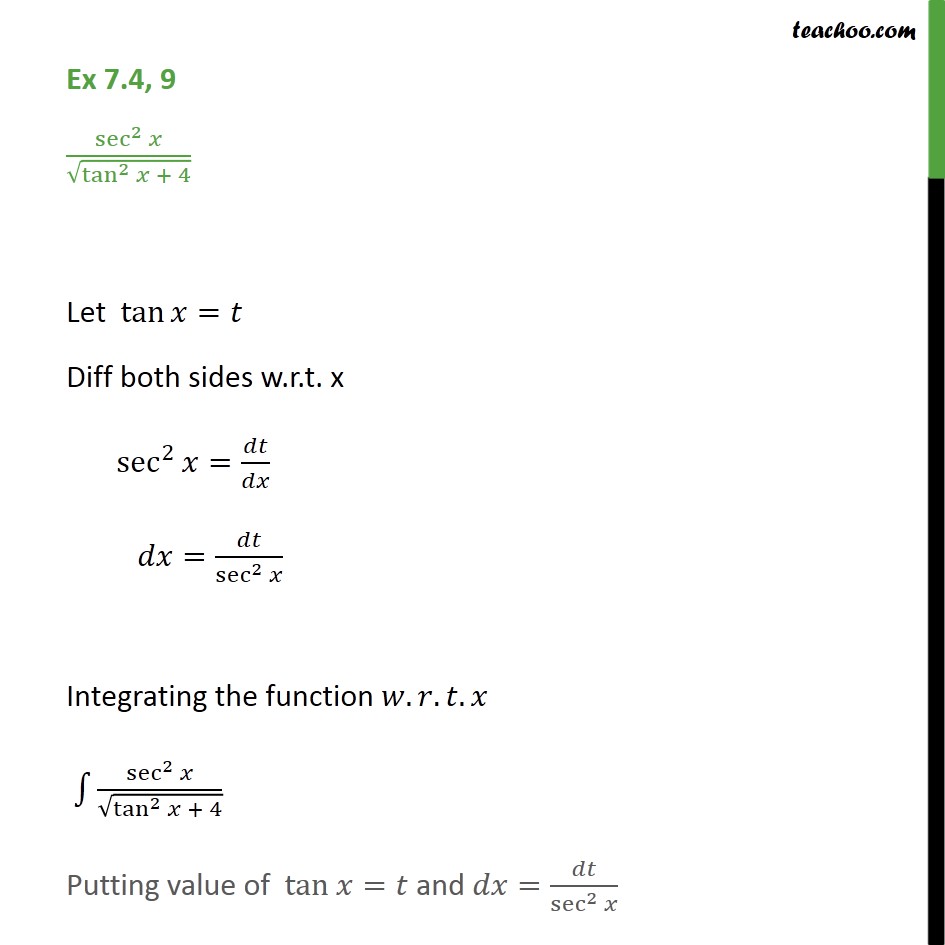
Ex 74, 9 sec 2 tan 2 4 Let tan = Diff both sides wrt x sec 2 = = sec 2 Integrating the function sec 2 tan 2 4 Putting value of tan = and = sec 2 = sec 2 tThe 2 in the given righthand side suggests that it should be one of the identities that involves a 2 somwhere And since your given righthand side involves only a close relative of cos x, I would choose cos ( 2 x) = 2 cos 2 ( x) for manipulation The manipulation can be done in various ways, but probably should use secCos 2x ≠ 2 cos x;

7 Techniques Of Integration Techniques Of Integration 7
What is sec^2x-tan^2x
What is sec^2x-tan^2x-Y tan2k1 x secnx dx y tan 2x k secn1 x sec x tan x dx tan2x sec2x 1 sec x m 2k 1 sec x tan x u tan x y tanmx 1 tan2x k1 sec2x dx y tanmx sec2kx dx y tanmx sec 2x k1 sec2x dx sec2x 1 tan2x tan x n 2k, k 2 sec2x y tanmx secnx dx x tanmx secnx dx 1 11 sec 11 2 9 sec 9 1 7 sec 7 C u11 11 2 u9 9 u7 7 C y u 2 1 2u6 du y u 10 2u8 u6 du y sec 2 1 2Start studying Calc exam formulas Learn vocabulary, terms, and more with flashcards, games, and other study tools




Integration Of Tan 2 X Sec 2 X Youtube
D is the differential operator, int is the integration operator, C is the constant of integration Identities tan x = sin x/cos x equation 1 cot x = cos x/sin x equation 2 sec x = 1/cos x equation 3 csc x = 1/sin x equation 4I'm currently stumped on proving the trig identity below $\tan(2x)\tan (x)=\frac{\tan (x)}{\cos(2x)}$ Or, alternatively written as $\tan(2x)\tan (x)=\tan (x)\secProve (sec^4xsec^2x) = (tan^4xtan^2x) Identities Pythagorean;
For each of the three trigonometric substitutions above we will verify that we can ignore the absolute value in each case when encountering a radical 🔗 For x = asinθ, x = a sin θ, the expression √a2 −x2 a 2 − x 2 becomes √a2−x2 = √a2−a2sin2θ= √a2(1−sin2θ)= a√cos2θ= acosθ = acosθ a 2 − x 2 = a 2 − a 2Inverse Trigonometric Formulas Trigonometry is a part of geometry, where we learn about the relationships between angles and sides of a rightangled triangleIn Class 11 and 12 Maths syllabus, you will come across a list of trigonometry formulas, based on the functions and ratios such as, sin, cos and tanSimilarly, we have learned about inverse trigonometry concepts also4 Chapter 10 Techniques of Integration EXAMPLE 1012 Evaluate Z sin6 xdx Use sin2 x = (1 − cos(2x))/2 to rewrite the function Z sin6 xdx = Z (sin2 x)3 dx = Z (1− cos2x)3 8 dx = 1 8 Z 1−3cos2x3cos2 2x− cos3 2xdx Now we have four integrals to evaluate Z 1dx = x and Z
Formula Used sec2x = 1 tan2x Calculation \\(\\frac{{{{\\left( {{{\\sec }^2}x {{\\sin }^2}x {{\\tan }^2}x{\\rm{\\;}}} \\right)}^2} {{\\cos }^4}x}}{{\\left {Tan 2x ≠ 2 tan xFirst, use the positive value of the ±
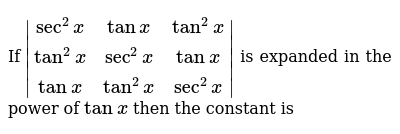



If Sec 2x Tanx Tan 2x Tan 2x Sec 2x Tanx Tanx Tan 2x Sec 2x Is Expanded In The Power Of Tanx Then The Constant Is
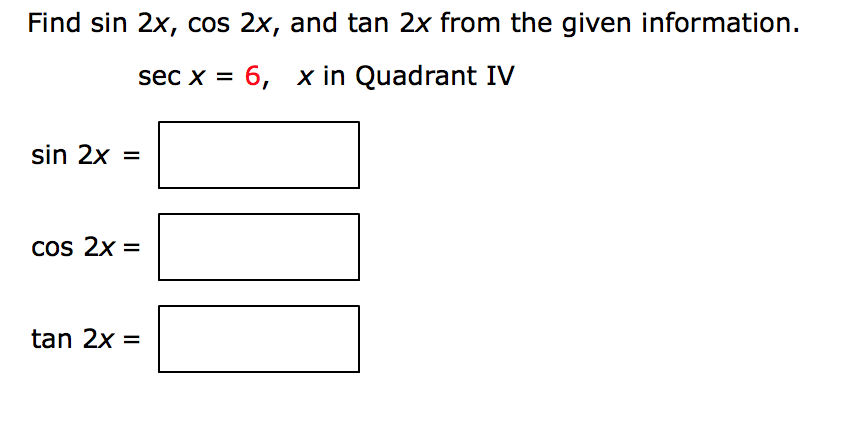



Find Sin 2x Cos 2x And Tan 2x From The Given Chegg Com
Tan 2x = 2 tan x/1 tan2 x = 2 cot x/ cot2 x 1 = 2/cot x – tan x tangent doubleangle identity can be accomplished by applying the same methods, instead use the sum identity for tangent, first • Note sin 2x ≠ 2 sin x;Tan 3x = 3tanx tan 3 x/1 3tan 2 x Trigonometry Formulas Sum and Product Identities Trigonometric formulas for sum or product identities are used to represent the sum of any two trigonometric functions in their product form, or viceversaWe have math\sec^{2}(x)/math math\Rightarrow \dfrac{d}{dx}\big(\sec^{2}(x)\big)/math This function can be differentiated using the "chain rule" Let




If Sec 2x Tan 2x 7 Then X



Geneseo Math 222 01 Trigonometric Integration
Safety How works Test new features Press Copyright Contact us CreatorsThe other four trigonometric functions (tan, cot, sec, csc) can be defined as quotients and reciprocals of sin and cos, except where zero occurs in the denominator It can be proved, for real arguments, that these definitions coincide with elementary geometric definitions if the argument is regarded as an angle given in radiansSecant, cosecant and cotangent, almost always written as sec, cosec and cot are trigonometric functions like sin, cos and tan sec x = 1 cos x cosec x = 1 sin x cot x = 1 = cos x tan x sin x Note, sec x is not the same as cos 1 x (sometimes written as arccos x) Remember, you cannot divide by zero and so these definitions are only valid




Tan2x Sec2x ただの悪魔の画像




Finding The Derivative Of Sec 2 X Video Lesson Transcript Study Com
If the power of the secant \(n\) is even, then using the identity \(1 {\tan ^2}x \) \(= {\sec ^2}x\) the secant function is expressed as the tangent function The factor \({\sec ^2}x\) is separated and used for transformation of the differentialAntiderivative of 2tan x sec x Compute tan x sec 2 x dx in two different ways a) By substituting u = tan x b) By substituting v = sec x c) Compare the two results Solution a) Compute tan x sec 2 x dx by substituting u = tan x If u = tan x 2then du = sec x dx and tan x sec 2 x dx = u du = 1 u2 c 2 = 1 tan2 x c 2To find the first solution tan ( x) = √ 3 3 tan ( x) = √ 3 3 Next, use the negative value of the ±




Cos 2x




Cos 2x
Trigonometric substitutions are a specific type of u u u substitutions and rely heavily upon techniques developed for those They use the key relations sin 2 x cos 2 x = 1 \sin^2x \cos^2x = 1 sin2 xcos2 x = 1, tan 2 x 1 = sec 2 x \tan^2x 1 = \sec^2x tan2 x 1 = sec2 x, and cot 2 x 1 = csc 2 xTan(x y) = (tan x tan y) / (1 tan x tan y) sin(2x) = 2 sin x cos x cos(2x) = cos ^2 (x) sin ^2 (x) = 2 cos ^2 (x) 1 = 1 2 sin ^2 (x) tan(2x) = 2 tan(x) / (1Proof of Integral of sec²x formula Take x as a variable, and it also represents angle of a right triangle According to trigonometry, the secant squared of angle x is written as sec 2 x in mathematical form The indefinite integration of secant squared function with respect to x is written mathematically as follows



Sec2x In Terms Of Tanx Trig Identity Youtube




Tan 2 X Tan 2 X 1 Cosec 2 X Sec 2 X Cosec 2 X 1 Sin 2 X Cos 2 X Brainly In
Start studying Calculus 2 Formulas Learn vocabulary, terms, and more with flashcards, games, and other study tools\∫\sec^n x\,dx=\frac{1}{n−1}\sec^{n−2}x\tan x\frac{n−2}{n−1}∫\sec^{n−2}x\,dx\ \∫\tan^n x\,dx=\frac{1}{n−1}\tan^{n−1}x−∫\tan^{n−2}x\,dx\ The first power reduction rule may be verified by applying integration by parts The second may be verified by following the strategy outlined for integrating odd powers of \(\tanThe cos2(2x) term is another trigonometric integral with an even power, requiring the powerreducing formula again The cos3(2x) term is a cosine function with an odd power, requiring a substitution as done before We integrate each in turn below cos3(2x) = cos2(2x)cos(2x) = (1 − sin2(2x))cos(2x)
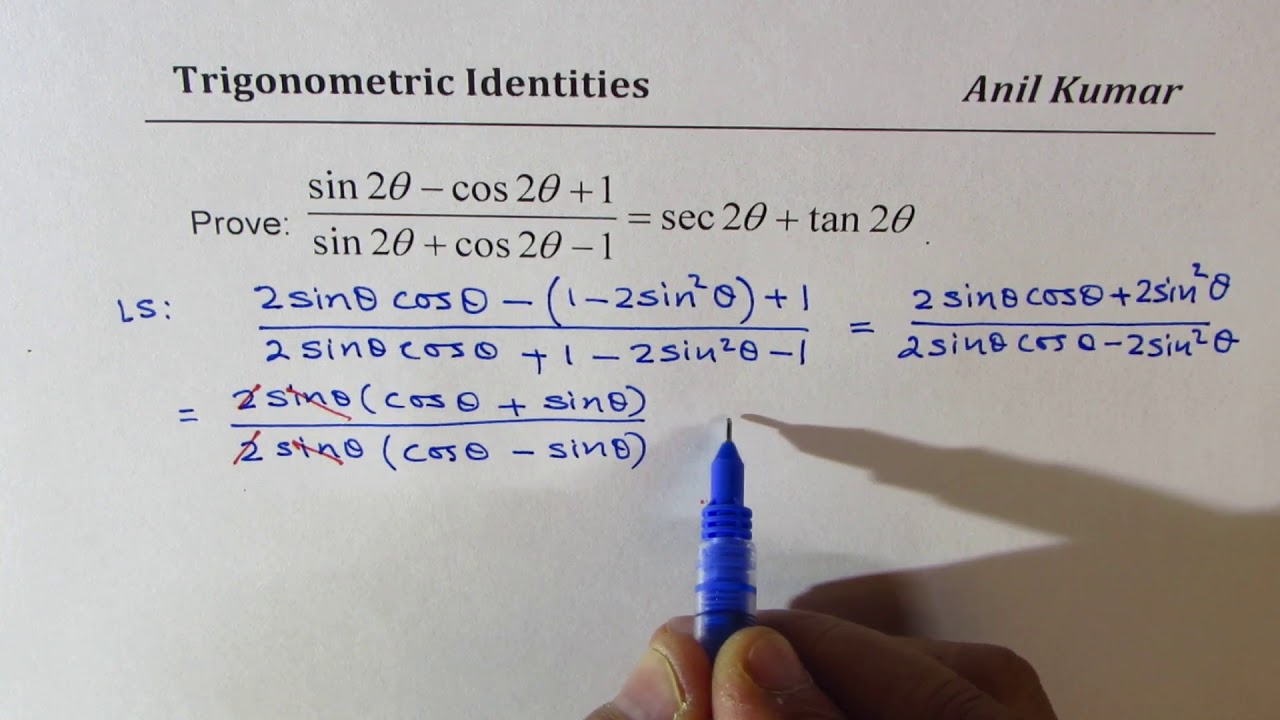



Trigonometric Identity In Double Angle Sin2x Cos2x 1 Sin2x Cos2x 1 Sec2x Tan2x Youtube




Integral Of Sec 2x Tan 2x Integral Example Youtube
True Start with the well known pythagorean identity sin^2x cos^2x = 1 This is readily derived directly from the definition of the basic trigonometric functions sin and cos and Pythagoras's Theorem Divide both side by cos^2x and we get sin^2x/cos^2x cos^2x/cos^2x = 1/cos^2x tan^2x 1 = sec^2x tan^2x = sec^2x 1 Confirming that the result is an identityTo integrate tan^22x, also written as ∫tan 2 2x dx, tan squared 2x, (tan2x)^2, and tan^2(2x), we start by utilising standard trig identities to change the form of the integral Our goal is to have sec 2 2x in the new form because there is a standard integration solution for that in formula booklets that we can use We recall the Pythagorean trig identity, and multiply the angles by 2Separate fractions Rewrite tan(x) tan ( x) in terms of sines and cosines Multiply by the reciprocal of the fraction to divide by sin(x) cos(x) sin ( x) cos ( x) Convert from cos(x) sin(x) cos ( x) sin ( x) to cot(x) cot ( x) Divide sec2(x) sec 2 ( x) by 1 1 Rewrite sec(x) sec (



Trigonometry Identity Tan 2 X 1 Sec 2 X Youtube



1
If k k is even and j j is odd, then use tan 2 x = sec 2 x − 1 tan 2 x = sec 2 x − 1 to express tan k x tan k x in terms of sec x sec x Use integration by parts to integrate odd powers of sec x sec xYou can check some important questions on trigonometry and trigonometry all formula from below 1 Find cos X and tan X if sin X = 2/3 2 In a given triangle LMN, with a right angle at M, LN MN = 30 cm and LM = 8 cm Calculate the values of sin L, cos L, and tan L 3\\int \tan^{2}x\sec{x} \, dx\ >




Sec 2x Formula Trigonometry Formula



Integrate Sec 2x Method 2
You may have some challenging algebra to simplify it Use the chain rule Let y(u) = ln u u(x) = sec x tan maths If Sin2x=3sin2y then prove 2tan(xy)= tan(xy) trig prove that the equation 2sin x cos x 4cos^2 x =1 may be written in the form of tan^2 x 2tan x 3=0Learn integral of square of secant function with introduction and proof for integration of sec²(x) rule with respect to x to prove ∫sec²xdx = tanxcDetailed explanation with examples on propertiesofinversetrigonometricfunctions helps you to understand easily , designed as per NCERT QnA , Notes &




Tan 2x 2tanx 5dy Dx 2 1 Tanx Sec 2x Differential Equations Brainly In




How To Show That Math Tan 2 X Sec 2 X 1 Math Quora
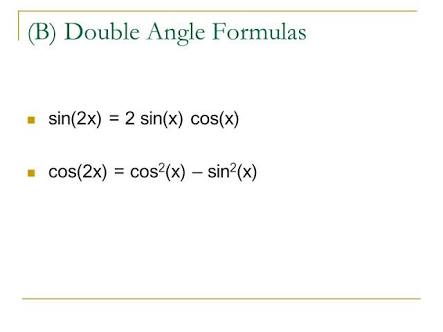
Solve your math problems using our free math solver with stepbystep solutions Our math solver supports basic math, prealgebra, algebra, trigonometry, calculus and moreLegend x and y are independent variables, ;Introduction to Tan double angle formula let's look at trigonometric formulae also called as the double angle formulae having double angles Derive Double Angle Formulae for Tan 2 Theta \(Tan 2x =\frac{2tan x}{1tan^{2}x} \) let's recall the addition formula



Tan 2x Sec 2x Youtube
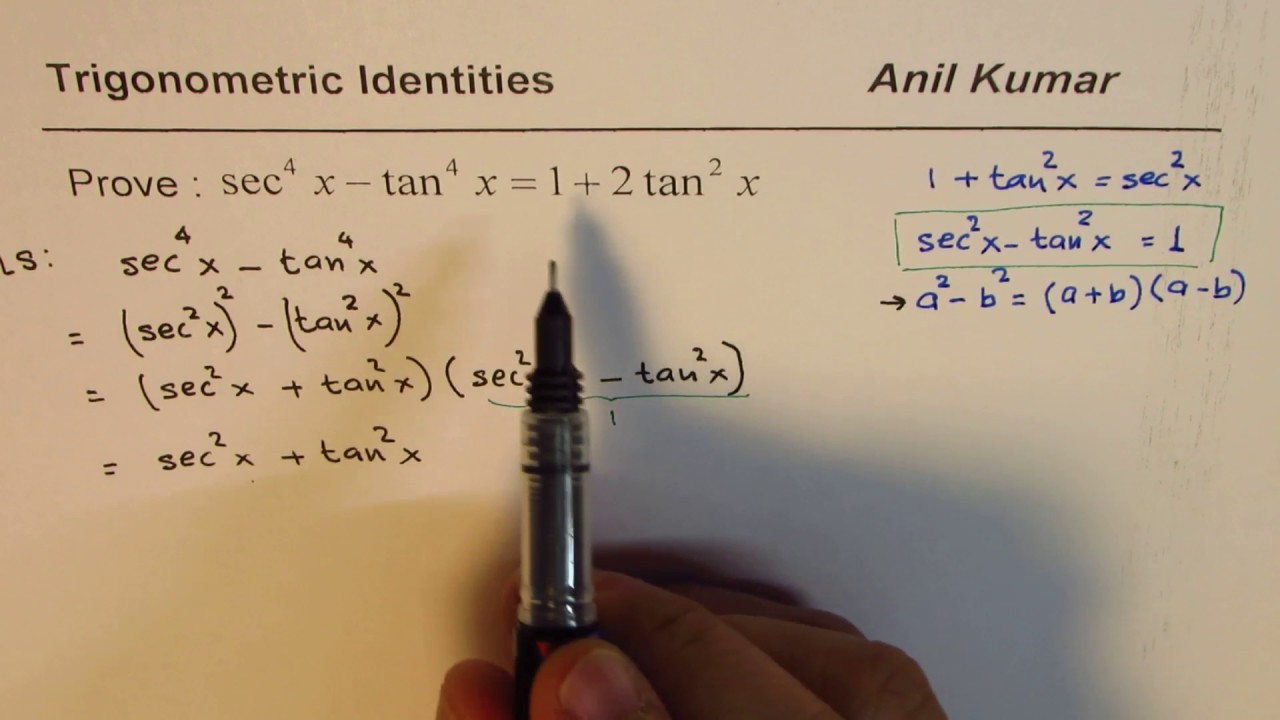



Trig Identity Sec 4x Tan 4x 1 2tan 2x Youtube
Get an answer for 'Prove that (tan x)' = sec^2 x' and find homework help for other Math questions at eNotes (tanx)' = sec^2 x Approved by eNotes Editorial Team Ask a Question Ask a QuestionGet an answer for 'solve for x sec^2x tanx3 = 0 2 2x^218=9y^2 3 x^2y^26x4y=3 4 25y^x^2=0 In 2,3,4 write the equation in standard form to identify the curve' and find homeworkIntegral of sec^3x https//wwwyoutubecom/watch?v=6XlSP58uisintegral of sec(x) https//wwwyoutubecom/watch?v=CChsIOlNAB8integral of tan




Tan 2x 1 Sec 2x Tan X




Question 12 1 Pts Dy Suppose Dx2 4 Sec 2x Tan 2x Chegg Com
Mathsecx tanx/math mathsecx = \dfrac{1}{cosx}/math And we know that mathcosx = \dfrac{1tan^2\frac{x}{2}}{1tan^2\frac{x}{2}}/math So, mathsecxWe can use the chain rule to find the derivative of 2sec 2 (2x) (bearing in mind that the derivative of sec^2 (x) is 2sec 2 (x)tan (x)) and it gives us a result of 8sec 2 (2x)tan (2x) ► The second derivative of tan (2x) is 8sec2(2x)tan (2x) ‹ Previous The Derivative of ln (7x) Next › The Derivative of ln (8x)It's other things too, like I'll do a problem times only to find out that there is no sine2x at all It's very frustrating From what I understand from having gone to a school that helped dyslexic and add or adhd kids, it seems somewhat similar, but as you say Mark it might not be dyslexia related at all



Solved Consider The Possible Identity Tan 2x Cos 2x 1 Cos 2x Sec 2x A State Any Non Permissible Values B Attempt To Verify Possible Identity Course Hero




What Is 1 Tan 2x
About Press Copyright Contact us Creators Advertise Developers Terms Privacy Policy &To find the second solution tan ( x) = √ 3 3 tan ( x) = − √ 3 3 The complete solution is the result of both the positive and negative portions of the solutionSolve your math problems using our free math solver with stepbystep solutions Our math solver supports basic math, prealgebra, algebra, trigonometry, calculus and more




Int Tanx Sec 2 X 1 Tan 2 X Dx




If Tan 2 X Sec X A 0 Has Atleast One Solution Then Complete Set Of V Askiitians
In mathematics, trigonometric substitution is the substitution of trigonometric functions for other expressions In calculus, trigonometric substitution is a technique for evaluating integralsMoreover, one may use the trigonometric identities to simplify certain integrals containing radical expressions Like other methods of integration by substitution, when evaluating a definite integral, itTan^2 x1=sec^2x So to get 1 on the other side of the equal sign wouldn't it be sec^2xtan^2x=1?



A 90 B 60 C 45 D Bo 1f X 4 Gauthmath



Tan2x Sec2x ただの悪魔の画像



Q Tbn And9gcr2jamezvnschsup9wyxdzj0wc0knie4dqfphj6okqiboct8vn1 Usqp Cau




7 Techniques Of Integration Techniques Of Integration 7




The Derivative Of Sec 2 X With Respect To Tan 2 X Is




1 Tan1 1 Tan2 1 Tan3 1 Tan45 2 N Brainly In Ryad Antonio Ebooks En Ligne Gratuit




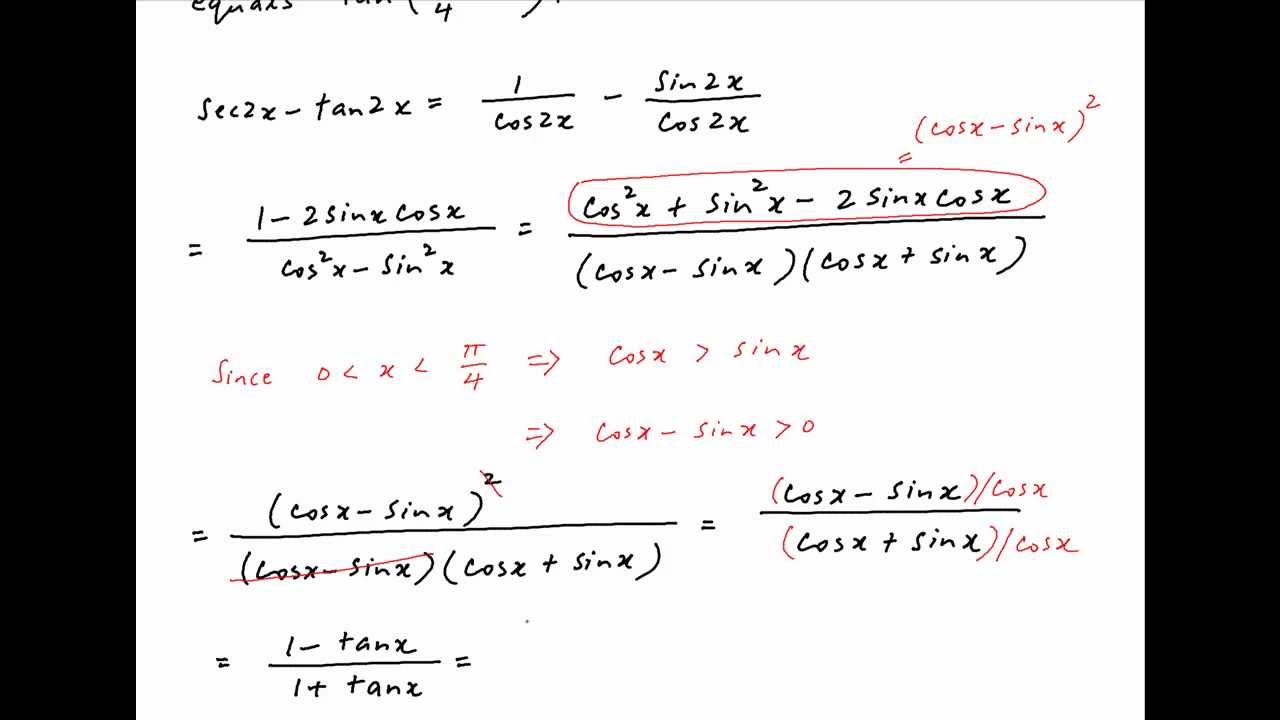
How Do You Prove Sec 2x Tan 2x Cos 2x Sin 2x Socratic




How Many Can You Derive From First Principles Ppt Download




Ex 9 6 5 Find General Solution Cos2 X Dy Dx Y Tan X



Tan2x ただの悪魔の画像




Find The General Solution For Each Of The Following Equations Sec2 2x 1 Tan 2x Mathematics Shaalaa Com




Integral 1 Tan 2 X Sec 2 X Youtube



What Is The Formula Of Tan2x Quora
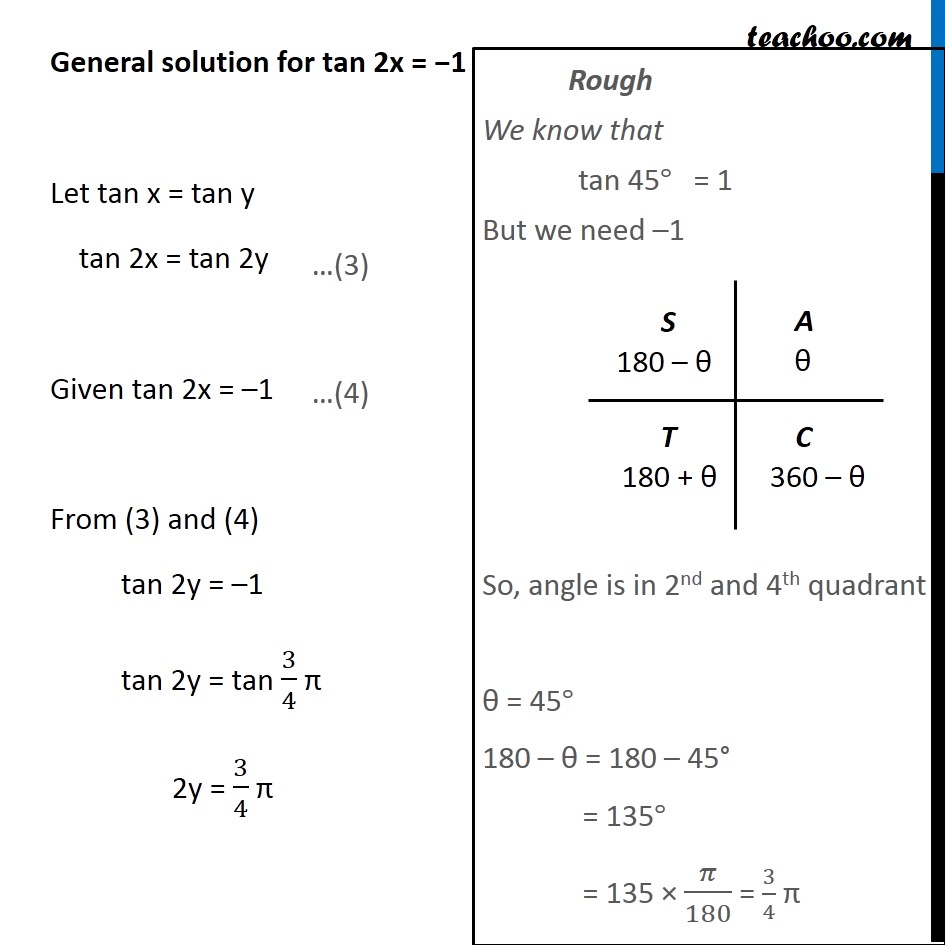



Ex 3 4 8 Find General Solution Of Sec 2 2x 1 Tan 2x Teachoo




Find The Derivative Of The Given Function Y Tan 2x 1 Cot 2x I Tried Converting The Original Function In Terms Of Sin And Cos But It Was Still Too Complicated To Be Called Simplified



What Is The Formula Of Tan2x Quora




How To Show That Math Tan 2 X Sec 2 X 1 Math Quora




1 Tan1 1 Tan2 1 Tan3 1 Tan45 2 N Brainly In Ryad Antonio Ebooks En Ligne Gratuit




Tan2x ただの悪魔の画像




Ex 3 4 8 Find General Solution Of Sec 2 2x 1 Tan 2x Teachoo




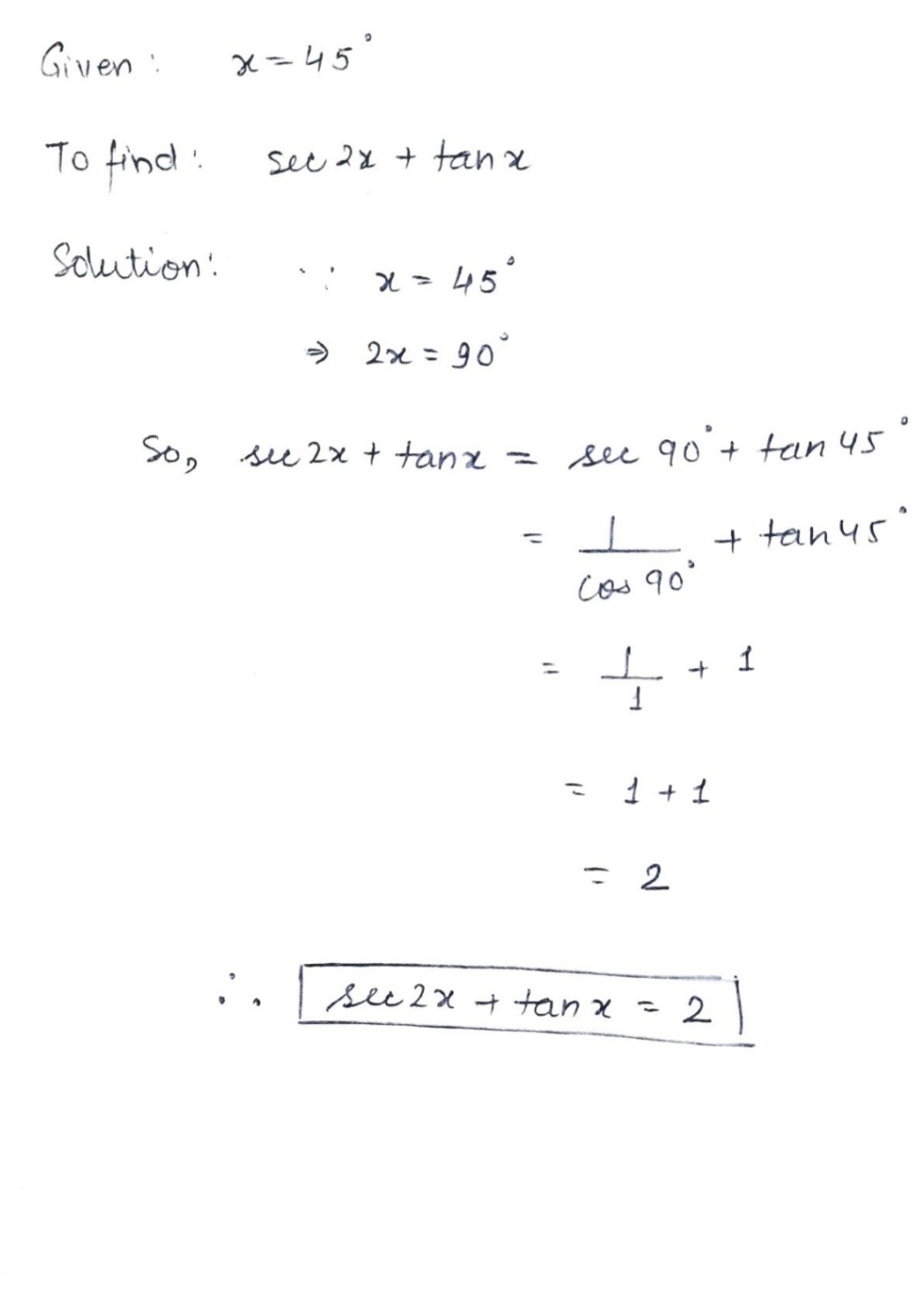
2 If X 45 Then Sec 2x Tan X Is Equal To Gauthmath




Ex 7 3 15 Integrate Tan3 2x Sec 2x Class 12 Ncert Ex 7 3




Tangent Half Angle Formula Wikipedia



Prove The Identity Tan P 4 X Tan P 4 X 2 Sec 2x Sarthaks Econnect Largest Online Education Community




The Most General Solutions Of The Equation Sec 2x Sqrt 2 1 Tan 2x Are Given By




Differentiate The Following From First Principle Tan 2x




Consider The Following Equations 1 Cosec 2x Sec 2x Cosec 2xsec 2x 2 Sec 2x Tan 2x Sec 2xtan 2x 3 Cosec 2x Tan 2x Cot 2x



F4ntussz Z2tvm




Answered 3 1 Tanx Tan 2x Sec 2x S Bartleby




Math34 Trigonometric Formulas




Cos2x Sin2x 1 1 Tan2x Sec2x Cot2x 1 Csc2x Cofunction




Integrate Sec 2x Method 2




Tan2x Sec2x ただの悪魔の画像




Proof Tan 2 1 Sec 2 Youtube




Integrate Sec 2x Method 2




What Is Integral Of Sin2x Tan2x Quora



bestpictjcry Tan 2x Tan 2x




Sec 2 X Cosec 2x Tan X Cot X Prove It Brainly In




X Y X Y X Y X Y Ppt Download




1st 3 Ways Verify Identity Tan 4 X Sec 2 X Tan 2 X 1 1 Youtube




Integration Of Tan 2 X Sec 2 X Youtube




How Do You Solve 1 Tan 2x 6 2sec 2x Socratic




Ex 3 4 8 Find General Solution Of Sec 2 2x 1 Tan 2x Teachoo



Integral Sec2x Tan2x Dx Youtube




The Derivative Of Tan X Is Sec 2 X Why Mathematics Stack Exchange




Trig Identity Sec2x Minus Tan2x T10 Youtube



The Derivative Of Tan 2x Derivativeit




Int 4sec 2xtanx Sec 2x Tan 2x Dx Log 1 F X C



Prove The Identity Tan P 4 X Tan P 4 X 2 Sec 2x Sarthaks Econnect Largest Online Education Community




Evaluating Trig Integral Int Tan 3x Sec 2x Dx Mathematics Stack Exchange




How Many Can You Derive From First Principles Ppt Download
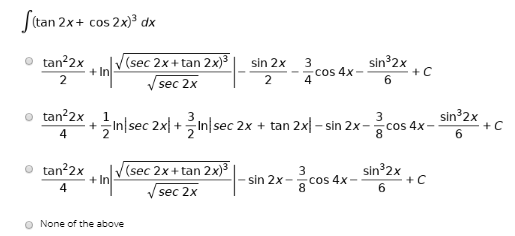



Answered Stan 2x Cos 2x Dx Tan 2x 2 V Sec 2x Bartleby




If 0 Lt X Lt Pi 4 Then Sec 2x Tan 2x
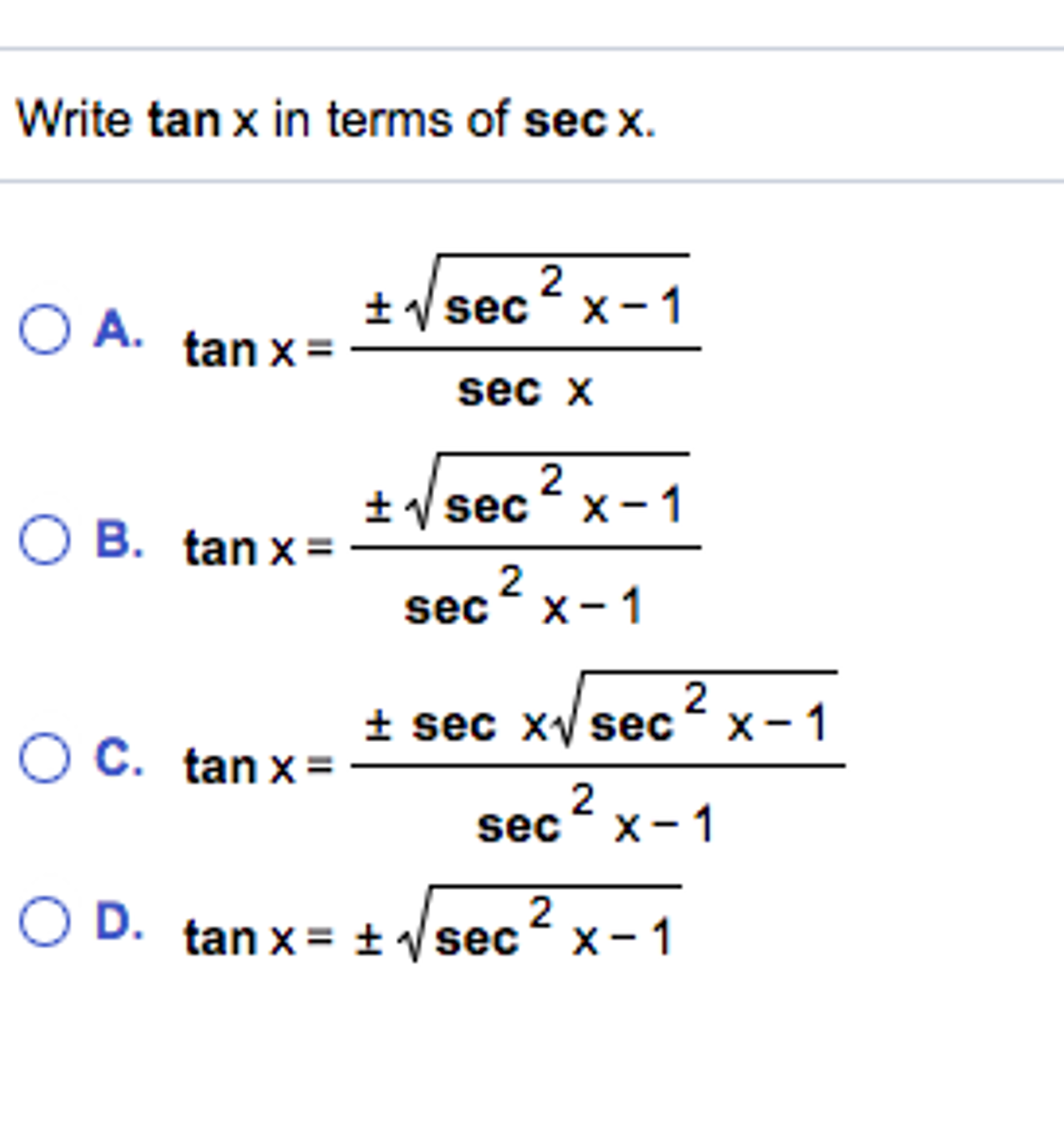



Write Tan X In Terms Of Sec X Tan X Plusminus Chegg Com



How Do You Find A Double Angle Formula For Sec 2x In Terms Of Only Csc X And Sec X Socratic




How Do You Simplify Sec 2x Tan 2x Sec 2x Socratic




2 Tan X 1 Tan 2 X Wyzant Ask An Expert Liana Charlne Ebooks En Ligne
.JPG)



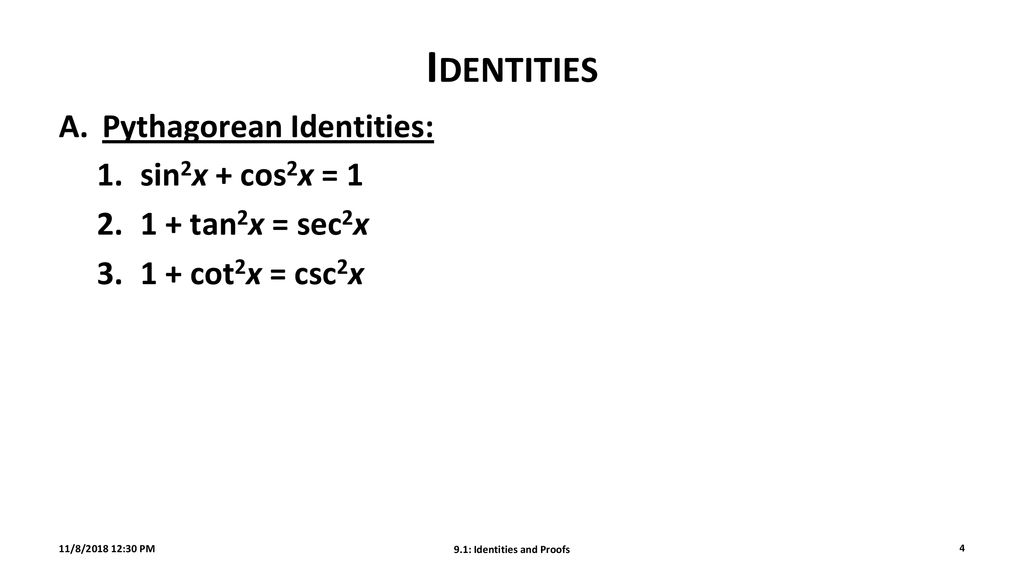
Every Day I M Calculatin I D3 Unit Q Pythagorean Identities
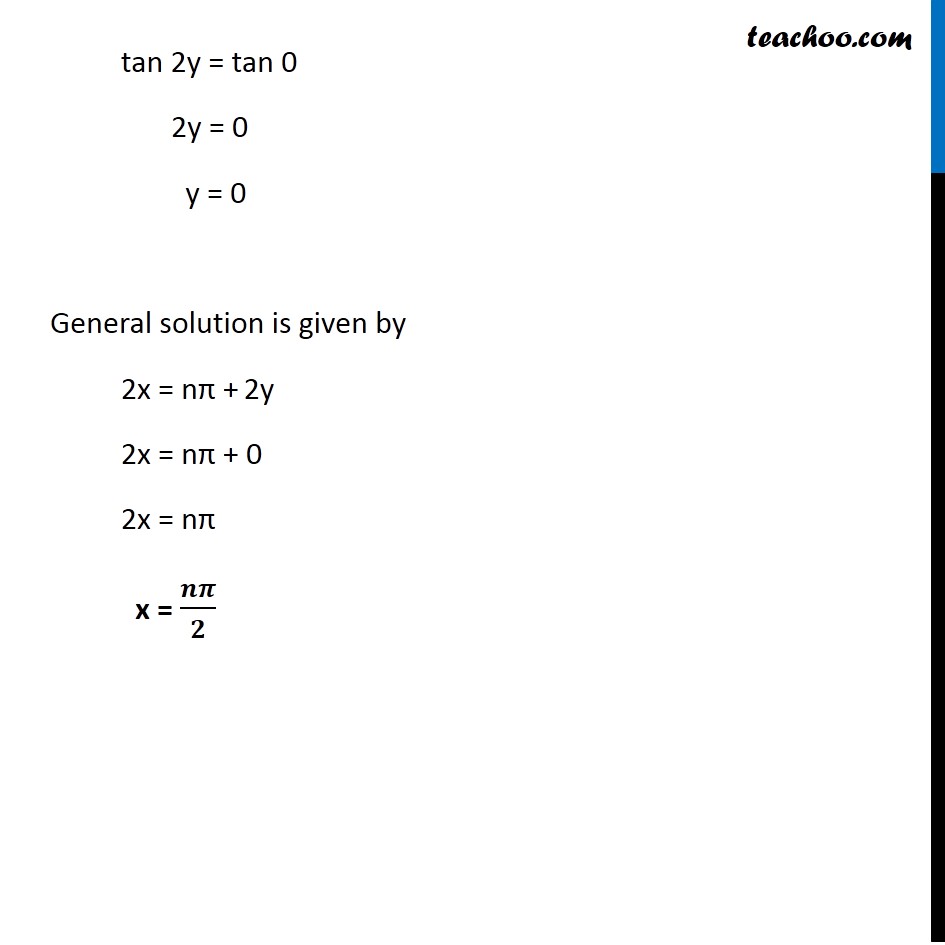



Ex 3 4 8 Find General Solution Of Sec 2 2x 1 Tan 2x Teachoo




Integrate Sec 2x Method 1




Sec 6x Tan 6x 1 2 Tan 2x Sec 2x Important Difficult Trigonometric Identity Youtube




4 Tan 2x 2 Sec 2x 1 0 X In 0



1



Prove Sin2x 2tanx 1 Tan 2x Socratic




Evaluate Int Sec 2xtanx Sec 2x Tan 2x Dx
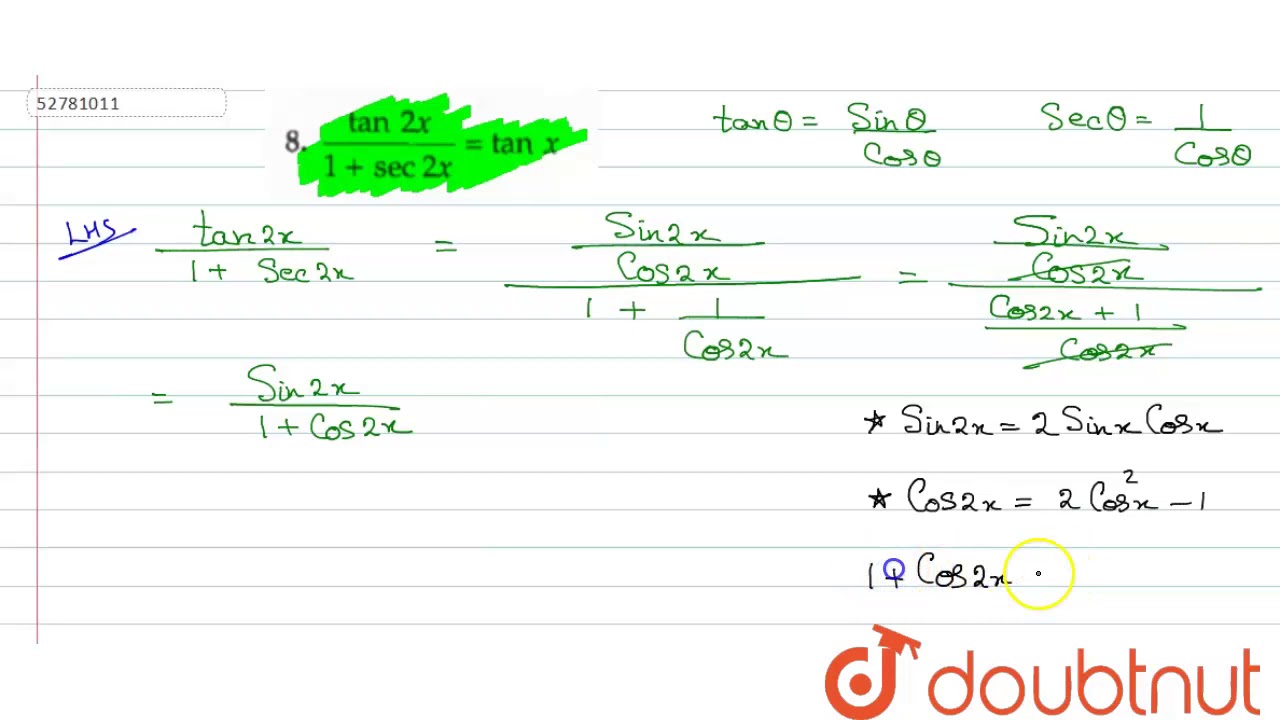



Tan 2x 1 Sec 2x Tan X Sin X Cos X 2 1 Sin 2x Youtube
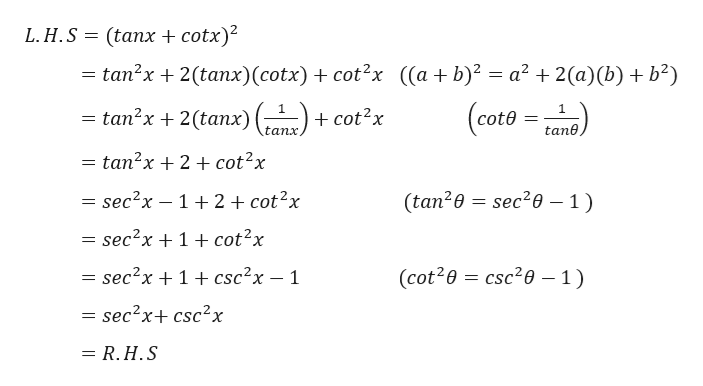



Answered The Question Says To Confirm That The Bartleby




If Sec 2x Tanx Tan 2x Tan 2x Sec 2x Tanx Tanx Tan 2x Sec 2x Is Expanded In The Power Of Tanx Then The Constant Is



What Is The Formula Of Tan2x Quora



Tan2x ただの悪魔の画像




Trigonometric Identity With Pythagorens Sec 2x Sin 2x Cos 2x Tan 2x Youtube




Finding The Derivative Of Sec 2 X Video Lesson Transcript Study Com



1



Integrate Sec 2x Method 2



Http Pue Kar Nic In Pue Pdf Files Recogn Ipu Iiqb 35 Ch7a Pdf



Ex 7 4 9 Integrate Sec 2 X Root Tan 2 X 4 Ncert Class 12
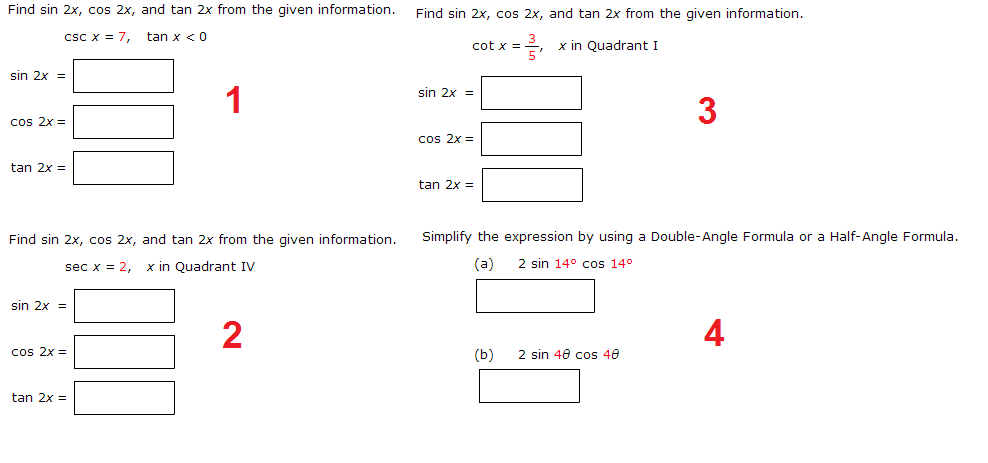



Find Sin 2x Cos 2x And Tan 2x From The Given Chegg Com




Tan2x ただの悪魔の画像



0 件のコメント:
コメントを投稿